Anne Jefferson at Highly Allochthonous has a good blog roundup on some of the events.
Chris Rowan (also of Highly Allochthonous) has a good rundown on the continued aftershocks of the Sendai Quake. He's also got a description of the kinematics of the quake. It's especially nice if you're trying to rectify those "beach balls" with the crustal block diagrams...
Callan Bentley's Mountain Beltway has a good post highlighting the GPS displacement vectors (distance and direction of motion) from Japan. He also provided a great initial summary of the quake.
Jessica Ball at Magma Cum Laude (one of my favorite blog titles) describes a few reasons why there are tenuous links between earthquakes and eruptions.
As I said earlier: it's like trying to pop a zit on your forehead by clenching your buttocks.
But back to the Sendai Earthquake. How to use this as a "teachable moment?" First, we can provide ways to help monetarily (through the Red Cross or similar) - although I would argue that Haiti is still worse off because of their lack of resources and infrastructure to help themselves. We can also educate people about earthquakes - perhaps by providing educated citizens, we can all benefit from better policies and systems in the future (hear that, gov. Walker?). Not just the dangers and hazards, but their underlying mechanics - how do they "work?"
So let's break things down a little bit. What are the forces at work here? We have tectonic forces, pushing the pacific plate beneath the island arc of Japan. The weight of the island arc pushes against the subducting pacific plate and creates a resisting, friction force that opposes the tectonic force driving the plates. When all forces are equal, nothing moves. As long as the friction force opposes the tectonic force, the plates will not move. But eventually, the applied tectonic force exceeds the friction force, and the plates move. This movement shakes the crust and releases energy (seismic waves) that travels through the earth. The more sudden and longer the movement, the stronger the shaking/earthquake. We can simplify this with a "free body diagram" of the forces involved (greatly simplified, but a decent first approximation).

To simplify this further, and bring the mechanics of the system into the classroom, there are several designs that use a brick on a spring. Pulling on the spring applies a tension force on the brick, but the brick does not move until the tension exceeds the friction force between the brick and the table (or sandpaper, or whatever). The forces are oriented in the same direction. Technically, the more analogous setup would be to have a compression spring, driven by a screw mechanism, since tectonic forces are more often compressive forces, not tension (but the direction and elastic behavior of the spring is still reasonably close).

The friction force is determined by the "Normal Force" (FN), which acts perpendicular (normal) to the surface and a "coefficient of friction." The greater the normal force, the harder the object is to move (try moving a refrigerator - and then try moving the box the fridge was shipped in). In addition, the coefficient of friction is a dimensionless number that describes how easy or hard it is to slide to objects past one another. Ice has a low coefficient, sandpaper has a relatively high coefficient.
For our "Earthquake Machine," the friction is provided by the brick and the sandpaper. The tectonic force is applied by pulling on the string (which is attached to the spring). The spring transfers the tension to the brick. Once the "tectonic" force exceeds the friction force the brick will slip forward - but only a short distance. The friction causes the brick to slow down and the applied force drops. The spring accumulates some of the tension by deforming (strain), then the accumulated strain can be released. When the spring's force diminishes to that less than the friction force, the brick stops moving; it sticks. The strain on the spring increases again until another slip. This is often referred to stick-slip behavior and is why many active faults don't shake all the time.
Here's the video:
Earthquake Machine Demo from Matt Kuchta on Vimeo.
I named mine "El Temblor!" I need to find some images of mexican wrestlers to paste on the sides and the brick to liven it up, I think. I designed mine to be easy to watch the brick, simple to construct, and cheap. Sort of a minimalist Earthquake Machine that I then loaded up with electronic sensors to graph some data (it's not SCIENCE until you graph some of the data...).
Let's look more closely at the force and acceleration data for one stick-slip set:
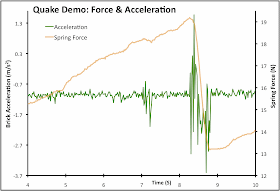
I was trying to keep my stress rate (winding up the spool) constant. You can see that the tension force (orange line) increases until the brick slips - releasing a bunch of accumulated tension stress. As the stress is released, the brick accelerates forward (green line). The brick slows down due to friction, which is why the acceleration graph goes into negative values. By adding a few data-logging tools (LoggerPro materials in this case), we can better see the magnitude of changes in the system. Plus, we now have a way of understanding what geologists are measuring before, during, and after earthquakes.
For a seismologist (the earthquake-studier), we can estimate the stress acting on rocks by measuring the relative movement between to pieces of crust. Places where the crust is moving tells us the stress is not accumulating. A lack of movement between plates of crust tells us the stress is being stored up (winding up the spring, so to speak). How much stress is accumulated can be estimated by the behavior of the rocks themselves (the strength of the spring) and the movement that should have occurred (how much the spring is deformed).
We don't know exactly when the crust will move, but we can provide a reasonable estimate of how strong the likely earthquake will be when it does move. If we're really lucky, we have hundreds (or thousands) of years of data that tells us how frequent earthquakes are in the region. So we may not know the hour, day, or even year - but we can at least point to areas that have a greater potential to move within a few decades or so. Given that cities are designed to last for many years, this is a pretty good estimate of risk for any particular city. Those governments with the capability and motivation to do so can plan for these earthquakes by mandating building codes, proper engineering, planning for disasters and so on. Japan's building codes likely saved tens of thousands of lives last friday.
So how can we apply our model to other situations? Take a look at this graph:
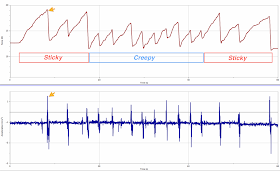
There are distinct periods of "stick" and "slip." The maximum stress accumulated was relatively constant, although there were a few slips near the beginning and end that were bigger than those in the middle. The friction was greater at the beginning and again at the end. So we have stick-slip movement here, while it more-or-less "creeped" along for part of the middle.
Here's another image:

Here we have another pattern. Mostly medium-sized slips until the very end. In the real world, these slips might represent the historical record of a medium-ish earthquake every century or so, and then a very big quake (arrow). Looking at the historical record, seismologists might decide that the "normal" pattern of medium-sized quakes had ceased and something was sticking more than it had been - making a stronger earthquake more likely. Note also the small accelerations (quakes) that don't interrupt the general increase in accumulated stress - not every quake reduces the possibility for another one.
That's not to say the big quake was "overdue." While our understanding of the model is very good, there may be other factors - perhaps the stress was being relieved somewhere else. Or perhaps the rocks aren't strong enough to store that much elastic energy. But it is never overdue.
Earthquakes are like wizards - "they arrive precisely when they mean to."
So what is the "spring" in our model? The rock itself. Rock has some elastic characteristics that allow it to store energy and then release it when the strength of the material is exceeded. I blogged about the elastic rebound theory earlier by using spaghetti noodles.
Breaking Spaghetti from Matt Kuchta on Vimeo.
Update for Steve:
Here's a link to the pasta mechanics post. (PS - I'm probably going to write another "geomorphically correct art" post this week)
UPDATE 2: Thanks for the pickup from Boing Boing and Vernier.
For those of you who are interested, the equipment was the low-g Accelerator and the dual-range force sensor. I'm not sure who else makes comparable equipment, but you could also rig up a standard spring scale and use some kind of "wobbly" thing on the brick (like a thin wire, or cup of water) to show the magnitude of movement.
Nice! Helps me understand quakes a lot better. I like the bringing together of physics and geology too.
ReplyDeleteThanks, Matt. How about a link to the spaghetti post? This is very good stuff for us non hard-rock people, and the physical modeling is awesome, thanks!
ReplyDeleteThanks - geophysics is a tricky language to translate into general physics and general geology at the same time.
ReplyDelete@Steve,
As a paleobiologist, I work though some of this stuff just to give me a chance to better understand it, too.
Great Demo. I was reminded of the bow and string interaction on a violin, where that same stick slip pattern can be observed. Here is slow motion footage of a violin and bow.
ReplyDeletehttp://www.youtube.com/watch?v=FO5bq6x_Tws
In the violin the resonance of the string makes the stick slip pattern much more regular and predictable.
yes hello i am wondering if you could help to demonstrate the deposit of sedimentary materials at the seam by installing a squeegee at the far end of the sandpaper.
ReplyDelete@hapa,
ReplyDeleteYou might be able to simulate an Accretionary Wedge this way - getting the "sediment" to accumulate properly would take some experimenting.
one thing i have used for similar purposes, and eaten, is nut butter.
ReplyDeleteDo you move the ratchet manually? Or have a sophisticated electronic gadget to make it move?
ReplyDeleteThis version I have to move the ratchet manually, but one could easily hook up a crank handle or motor to make things easier. I like the audio-visual cues students get from the click-click of the ratchet noise.
ReplyDelete